The Celestial Sky: A Coordinate System

Motion has no meaning with out a coordinate system. Indeed, physics can't be described without a coordinate system and measurements don't mean anything without a coordinate system. Events don't occur without a coordinate system because an event is something that occurs at a specific time in a specific place.

For instance, if you text message your friend the following:

Meet me then nothing happens.

If you specify a coordinate:

meet me @ *$

still nothing happens because you have not specified a time, only a location.

Hence our coordinate system for events consists of a location plus a time; otherwise known as spacetime.

---

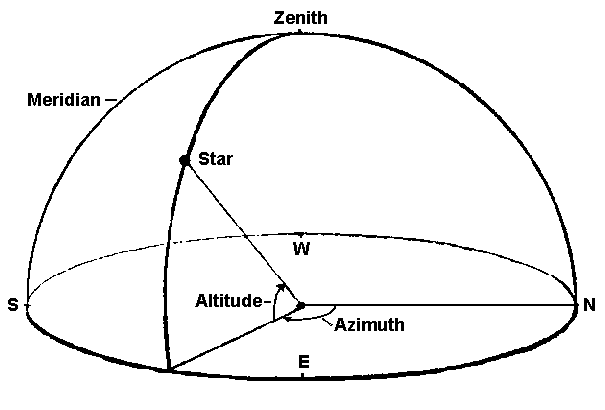
For an observer standing on the surface of the earth, the simplest possible coordinate system for observing the motion of a star is shown below:

• Directly overhead is the zenith

• a star will rise in the east and set in the west and at any given time it will have some height about the horizon (e.g. ground) which corresponds to the angle between the star and the hoizon. When the star is directly overhead, that angle would be 90 degrees. This angle can be called altitude.

• The initial rise point in the East is some number of degrees from north. A star that rises due east would have this azimuthal angle be 90 degrees. One that rises in the SE would be 135 degrees.

• The meridian is an imaginary NS line running through the zenith. As the star approachs the meridian it is still "rising"; at the meridian its at its highest altitude in the sky; as the star continues its motion it approaches the western horizon and moves away from the meridian and is thus setting.

• This model assumes that the earth is flat (with respect to the observer) and since the radius of the earth is a lot bigger than your height of 1.8 meters, this assumption is valid.

A slight improvement on this coordinate system would define the horizon as the equator of the earth and 90 degrees +/- from that horizon would define the poles of that coordinate system. This now assumes the Earth is a sphere! (most historical data indicates that, at the time of the Early Greeks, they did think the earth was round).

In this coordinate system, and observer at some latitude would observe that any star reaches a maximum altitude in the sky. That maximum altitude is equivalent to the latitude of the observer and is known as declination . Thus if you were on a sailing ship and had a list of declinations of brightstars and measured when those stars reached their maximum altitude, you would then know your latitude.

Knowing your longtitude, however, would require a universal time system on the Earth and that is not established and defined until the 19th century (1884 to be exact).

However, this coordinate system still can not explain why the amount of time the sun is in the sky varies.

A simple experiment done initally by chinese astronomers around 1000 BC gives a clue and all you need to do is put a stick in the ground:

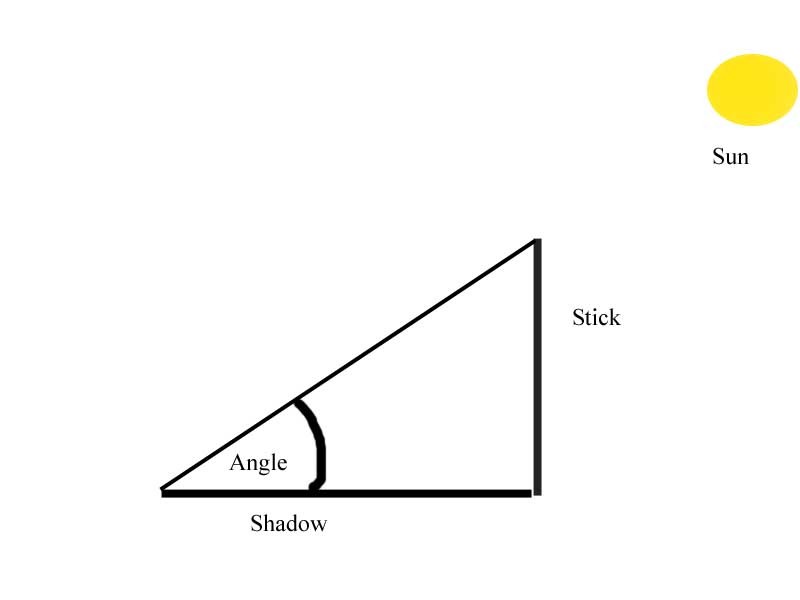
If you do this, you will notice that at solar noon (when the sun is at its highest point in the sky) there is a shadow of a certain length from which you can measure an angle. If you do this long enough (e.g. for one year) you will notice there was a maximum angle measured and a minimum angle measured (this works for any latitude).

The difference between those maximum and minimum angles will be 47 degrees. This difference is equal to twice the "tilt" of the Earth. Therefore the earth has a "tilt" of 23.5 degrees.

But a tilt with respect to what?

This is an important complication with respect to the idea that the Earth is at center of the solar system and the sun revolves around it. The tilt hypothesis directly allows for different amounts of time for the sun to appear in the sky but understanding tilt in the context of the geocentric model is hard (and likely, therefore was ignored).

The correct understanding of tilt requires a different coordinate system

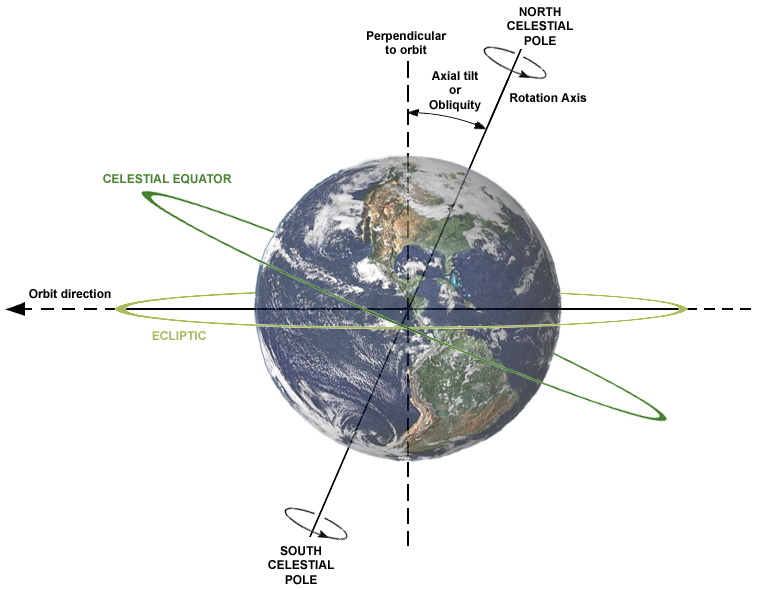
Which establishes the "horizon" as being an extension of the equation of the sun. This is known as the ecliptic plane and we will see later that all of the planets orbit within this plane. Thus the tilt of the earth means that its rotational axis is titled by 23.5 degrees with respect to the equator of the sun (or equivalently, the ecliptic plane).

So from the point of view of an observer on the ecliptic plane, the equator of the Earth is tilted by 23.5 degrees.

Since coordinate systems are often relative then they can be shifted. So from the point of view of the observer on the Earth, the ecliptic plane is tilted by 23.5 degrees relative to the Earth's equator:

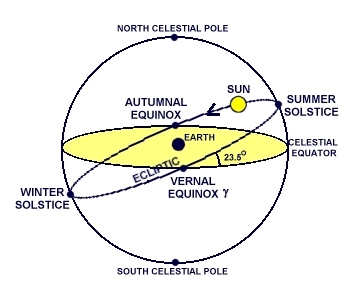
So this means that in the summer solstice the Sun has a declination of 23.5 degrees, meaning that if you were at 23.5 degrees the sun would be directly overhead (e.g 90 degress above the horizon) at noon.

Now finally, let's consider the case of Eugene, which is at a latitude of about 44.5 degrees.

• 44.5 - 23.5 = 21 degrees away from that reference point
• On the summer solistice the maximum elevation of the Sun above the horizon would therefore be 90-21 = 69 degrees.

• In the winter solistice, the sun would have a declination of -23.5 meaning that if you were at -23.5 degrees south latitude, the sun would be 90 degrees above the horizon. Eugene would be 44.5 - (-23.5) or 68 degrees away from that reference point -so on Dec 21 in Eugene the sun have a maximum angle above the horizon of 90-68 = 22 degrees.

• At the time of the equinoxes the sun has a declination of O degrees meaing its directly overhead at the equator or, in the Eugene sky, has a maximum altitude of 44.5 degrees above the horizon.