Hydroelectric Power Generation
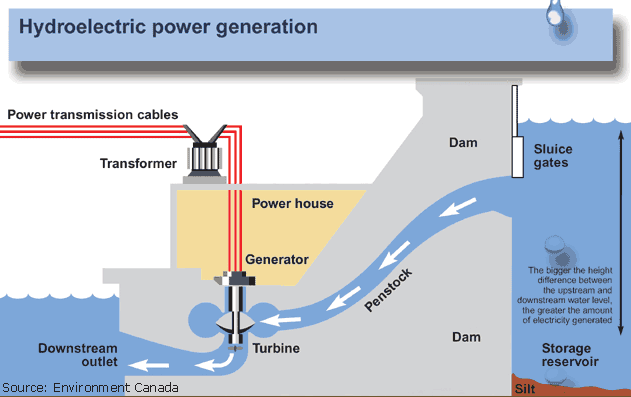
In more general terms, hydroelectric power represents the conversion of potential energy, in the form of water stored at some height H, above the turbine, into kinetic energy (the movement of the turbine).
The potential energy is given by the equation
Where m is the mass of water, g is the gravitational acceleration of the Earth (9.8 m/sec2) and H is the effective Height of the water (or the total vertical distance it will fall).
A large dam creates a head (H) which can then feed a system of turbines that would be located in a powerhouse:
The total power of any hydro system can be easily computed from the parameters of the system. Probably the best "engineering" short form for this expression would be
Example specs for a 1000 MW Nameplate Capacity Hydroelectric Dam
Overall system efficiencies depend upon flow rate, turbine design and head. In general, systems with the highest H are the most efficient. System efficiencies vary from 50-95% with most large scale hydro projects being around 90%.
Sources of energy loss are usually related to friction in the penstock flow or friction associated with turbine spinning. Very large turbines have so much mass/inertia that they are almost frictionless. For microhydro (see below) turbine design is the limiting factor on efficiency and is usually around 60%.
Example 1:
The Columbia River, in places, has an average flow of about 6000 cubic meters per second. What dam height (H) is needed to produce 1000 MW? (1000 MW = 1,000,000 KW)
Assume &eta = 0.9
Solve for H 
H = 1,000,000/(.9 x 9.8 x 6000) = 19 meters
Example 2:
The Colorado river has a flow of about 500 cubic meters per second. Again assuming &eta = 0.9 what is the value of H.
Well the flow is down by a factor of 12 so H has to go up by a factor of 12
228 m (about 750 feet).
Fun with numbers?
How long of coal train would you need to equivalently produce the annual energy output
of a 1000 MW Dam?
So,
In one year we will produce 1,000,000 KW x 8760 hrs = 8.76 x 109 KWH
That is equivalent to (1.2 x 106/.36 = 3.3 Million tons of Coal or 33,000 rail
cars or a 470 mile long coal train!
How many Goodyear Blimps of natural gas does this represent?
And away we go to get 1.7 x 1010 cubic meters or 2.8 million blimps
now that's a shitload of blimps.
These equivalencies manifest themselves as low operating costs for hydro:
and this is why it became the technology of choice.
Oh yeah, concrete.
Remember that exercise we did.
Well if we take all the concrete in the 58 dams in the PNW we get about 50 million cubic yards of concrete. Is that a lot?
Some equivalencies:
Example 1:
You own some land with a small creek on it that has a flow of 100 cubic feet per minute (.047 cubic meters per second).
The effective head of a typical "run-of-river" hydro installation is 10 feet (3.05 meters).
The working efficiency of your turbine is 60%
How much power will you generate:
P = 9.8 x 0.6 * .047 * 3.05 = 840 watts.
This shows the basic problem of microhydro.
Either you need a larger value for H, which is toplogically difficult for most locations, or you need a larger value for Q, which bring up various water rites issues.
Example 2:
Example 3: Mckenzie River (Leaburg dam)
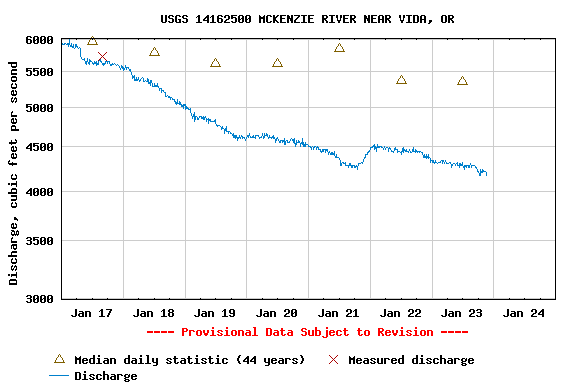
As a result of variations in Q and H throughout the northwest, there is considerable scale/range involved in hydropower plants.
