Density Dependent Consumption

Premise: the rate of prey consumption by a predator rises as prey density increases

But, what is the functional form of this rise and what are the limits? For instance, is it linear (Y = ax +b) are might there be other issues?

What might some of the variables be to consider:

Let's think about predators - what do they do?

• Look for and locate potential prey to consume
• Spend time (which we call "handling time") chasing, catching, killing, eating and digesting.

This handling time concept now limits a predators consumption rate, espcially if the handling time is large.

The total predator time is

T = T_search + T_handling

Now let's specify H_a as the amount of prey captured by the predator during time T (this would be an integer number). The little a refers to "attack".

Since handling time should be directly proportional to prey capture rate we have:

T_handling = H_aT_h

where T_h is the time spent on handling of 1 prey.

What is the capture strategy of a predator? Mostly random. A predator hunts around some area over some time interval and so there is a "search rate" parameter which we will call a which has units of area/time (e.g. meters²/seconds)

After searching for some time, T_search, a predator has covered an area of:

aT_search(meters²/seconds x seconds = meters²)

and has captured:

aHT_search

prey, where H is the prey density per unit area (N/area).

So,

H_a = aHT_search

or

aT_search = H_a/H

and

T_search = H_a/aH

Now one evaluates the total time budget

T = T_search + T_handling = H_aT_h + H_a/aH

We are interested in expressing H_a as a function of H and T.

1. T = H_a*(T_h + 1/aH)

2. rewrite T_h + 1/aH as aHT_h/aH + 1/aH or (aHT_h +1)/aH

3. we now have T = H_a(aHT_h +1)/aH

4. and then H_a = aHT / (1 + aHT_h)

for the asymptotic case where aHT_h >> 1 then H_a = constant = T/T_h

This equation has a functional form that looks like this:

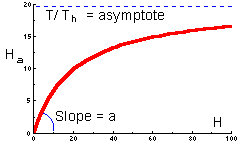
How does one interpret this?

• There is a specific amount of handling time (T_h) associated with each prey item eaten or attacked that is invariant of the density of the prey (H).

• Thus, while prey items are easier to find as their density increases, handling time per prey item is the same, and the maximum number of prey items eaten or attacked is determined by the ratio of total available searching time to handling time (T/T_h).

• The feedback is that as prey density increases (therefore decreasing search time), the proportion of total Time spent in the handling phase increases.

• Obviously when all available time is spent handling prey, you can only process/attack prey at a constant rate and hence the number of attacked prey levels out (reaches a plateau).

• Sharks behave like this to some extent which is why there can be plenty of food swimming around them