We begin with the heat loss or gain via phase change in water.

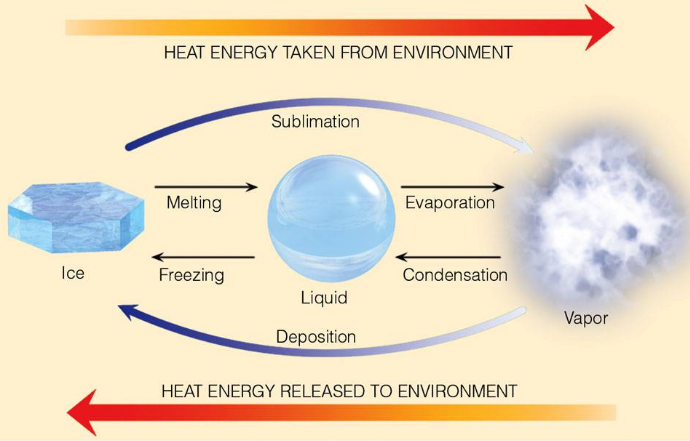
1. When a parcel of air expands (initially because of heating by the sun), it does work against the environment. But this is an adiabatic process so the parcel can not gain heat, but must lose internal energy in order to perform the work. This leads to a necessay temperature decrease.
   
   
2. As it rises and cools, the vapor pressure of water in equilibrium with liquid water decreases (this is the e/s_s process). Water vapor in excess of the equilibrium amount then condenses and forms the floating cloud of tiny water droplets. This releases heat to the environment . Note that this can lead to a runaway situation to be a driver of convective thunderstorms.
   
   
3. The formation of water vapor in a system is primarily due to evaporation which adds heat to that system (it takes away heat from the environment) and so the lapse rate of moist air is necessarily smaller than dry air.
   
   
4. Condensation of water vapor cools a system. Therefore a rising parcel of air which should cool, cools even further due to decreasing amounts of water vapor (some vapor condenses and that releases heat to the environment ). This "condensation" atlitude is usually reflected by constant altitude cloud bases.
   
   
5. If saturated air (moist air) is created near the surface it expands and rises at an initial rate given by the moist adiabtic rate; Γ_s in previous notation. It will continue to rise as long as conditional instablity holds:
   
   Γ_a > Γ > Γ_s
   
   
6. But this process of producing moist air and can occur at any altitude in the rise of an air parcel.
   
   
7. The amount of water vapor in a parcel of is any given time is usually less than that required to saturate the air. The relative humidity is the percent of saturation humidity.
   
   

Examples:

• At 20 C the saturation vapor density is 17.3 g/M³ (note the funny units of density).
• If the actual water vapor density is 10 then RH = 10/17.3 = 58%

If water vapor comprises 1% of the volume of a stationary piece air, how much does the temperature change compared if this was pefectly dry air?

• 1% means 1% of the molecules are H₂O
• 1% of the air is moist; 99% is drive
• Molecular width = .99*dry + .01*wet
• molecules = N₂ = 14; O₂ =16 ; H =1
• In our atmosphere N₂ = 78% ; O₂ = 21% (any idea on what makes up most of the remaining 1%?)
• .78*28 + .22*32 = 28.88 (28.97 is real answer)
• 28.97*.99 + 18*.01 = 28.86
• T₁/T = M_d/M_moist = 28.97/28.86 = 1.0038
• Take T = 288K ; T₁ = 1.0038*288 = 289.1 ; 1 deg correction
• Okay so why (physically) has the temperature gone up?
  
  
  
  

The Skew T Log P diagram has long been a valuable tool but full discussion of this is way time consuming and pretty boring. Suffice it to say, that this is data that shows you the dewpoint temperature as a function of pressure, which allows one to figure out what the atmospheric water vapor content is at variou surfaces. The modern form of this is the water vapor 500 MB map:



Suppose you find from a skew T -ln p chart that from 1000 to 950 mb that the amount of water vapor change is 0.25 g/kg. Then,



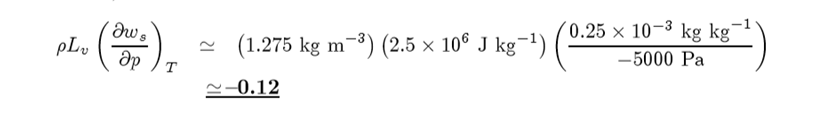
We are going to make use of this term, and the fact that it is small compared to 1 to derive and expression fo r the rate of change in temperature with heigh of a parcel of air undergoing a saturated adiabatic process. In other words, we will be deriving Γ_s but in a slight different manner than before to get:



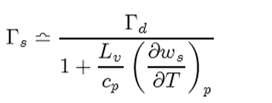
Again, this the denominator will be > 1, Γ_s < Γ results. In principe (δw_s/δT) is something that can be measured from the Skew T ln p diagram for various pressures.

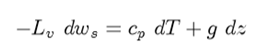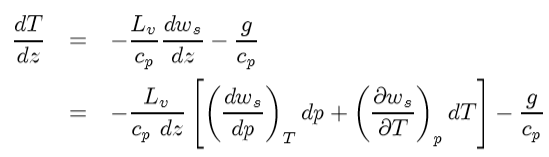
Starting as before: Divide both sides by c_pdz ; rearrange terms of isolate dT/dz and expand (dw_s/dz) in to pressure and temperature terms: Look, (dp/dz) showed up and you know what to do there. Hydrostatic equilibrium 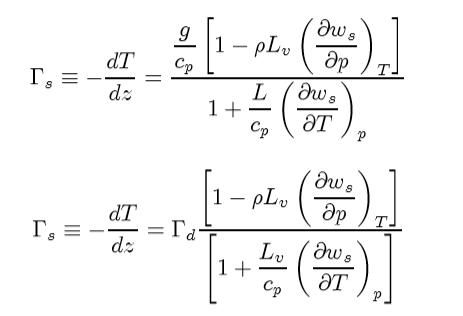Now above we showed that the second term in numerator in the above equation is essentially zero for a typical atmospheric case. So,

Physically, what is driving the correction term to the adiabatic lapse rate is the variation of available water as a function of local temperature. Remember, w_s is the saturation mixing ratio. When the dewpoint temperature is reached the air will be saturated and clouds will form.