Understanding Exponential Growth

Human population increases exponentially:

Now the representation of exponential growth as manifest by the rabbits is where a lot of unnecessary confusion comes into play when describing the population growth of humans. The term exponential growth does not immediately imply unbounded growth, and it is foolish to think otherwise. Period. In fact, virtually all mammalian populations grow according to a method called the logistic growth curve. The waveform of that kind of growth is shown below:

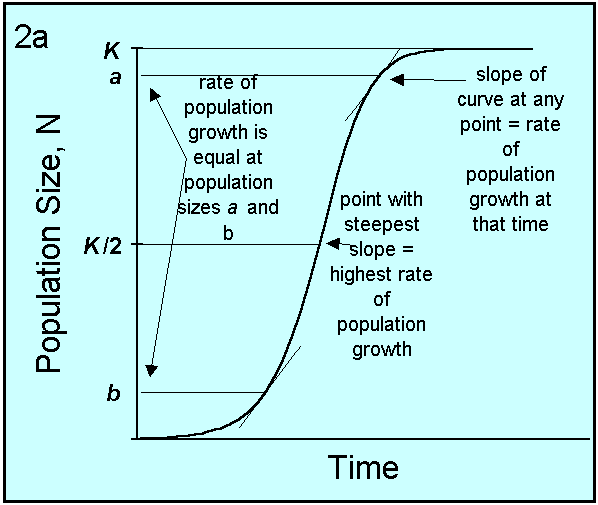
And exhibits the important feature that, as the population reaches its carrying capacity (K), the rate of growth decelerates.

Currently the rate of growth of the world's population is in the deceleration phase as evident by the following data: (however, this still means the population is growing exponential but at a rate which is changing).

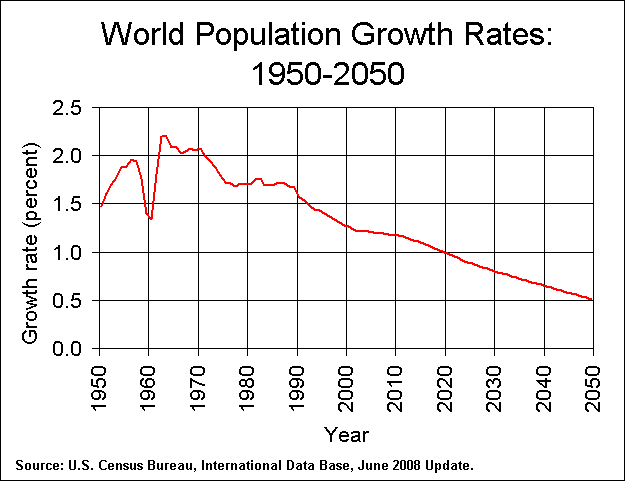
Some important features of this waveform are the following:

• The obvious dip in the 1958-1960 data was due to the Great Leap Forward in China. A combination of both natural disasters and decreased agricultural output caused China's death rate to rise sharply and its fertility rate to fall by almost half.
  
  
• Overall, however, the world population growth increased from 1.5 to 2.2 % in just a little over a decade. When we soon relate growth rates to doubling times, you will see the disastrous trajectory that humanity was on.
  
  
• This unsustainable growth rate was recognized and a massive UN led fertility education effort occurred on a global scale. This was fairly effective as the population growth rate would decline, over a timescale of 15 years, from 2.2% down to 1.7%.
  
  
• But, by 1977 the growth rate stabilized at around 1.7% for about another 15 years. In this period, human growth was truly increasing exponentially at constant growth rate.
  
  
• Starting around 1990, the growth rate begins to slowly decline. The rate of that decline over the period of 1990 to 2000 was .03% per year. Since 2000, the rate of decline has slowed dramatically to .01% per year.
  
  
• The current world growth rate is 1.12% per year.

Currently the world's population is growing at the rate of 1.12% per year, down significantly from the growth rate 30 years ago. But, small differences in growth rates make big differences in the future population that as ultimately the exponential doubling time is what matters.

Right click to open this example in a new window and do the following:

• Set the growth rate to its current value of 1.12 and then set the year to 2050 (you will still be alive then). Note the value (should be around 10.5 billion).
  
  
• Now assume continual decreasing of the growth rate at the rate of about 0.01% per year by changing the rate to 0.78 (average rate under this scenario) and note the value. Small differences in rates to seem to matter.
  
  
• There are some theories that suggest the world population growth rate can never get below 1%. Set the rate to 1% and the year to 2100. Note the value!
  
  
• Suppose you want to live in a world where the population is stabilized at 8 billion in the year 2050. Turn the year back to 2050 and adjust the growth rate to yield that outcome. How many times lower is this rate than the current rate (1.12%)? Do you think this is a likely scenario?

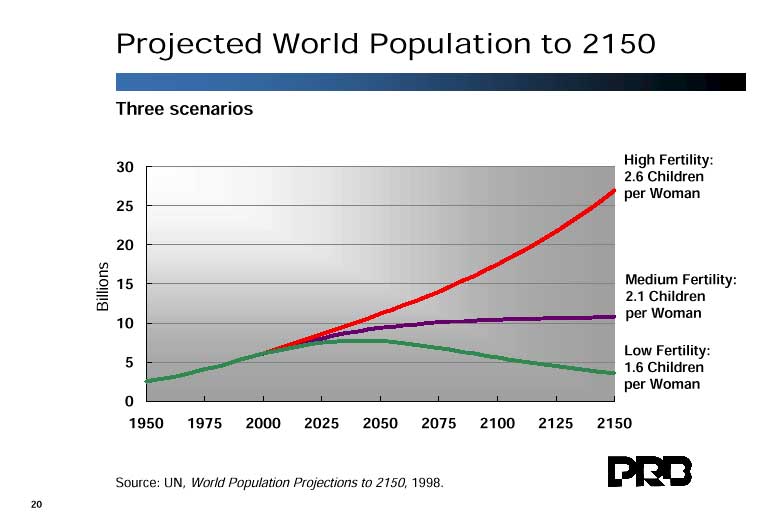
Future population predictions are difficult to make (and will be covered later in this course). It is more complicated that the simple approaches that produce these alternatives: