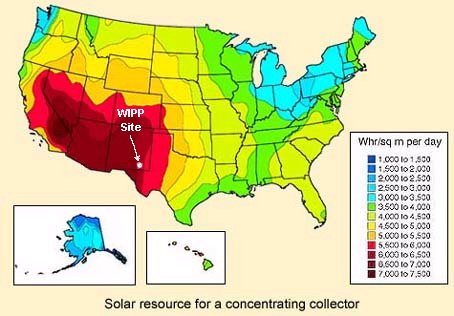
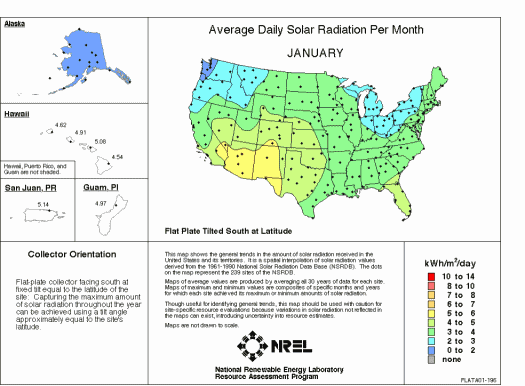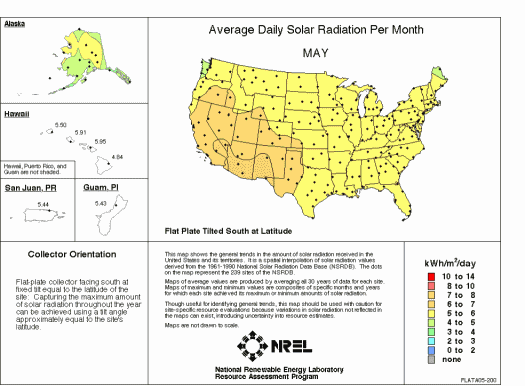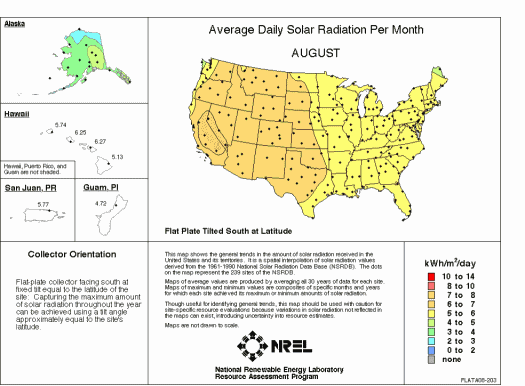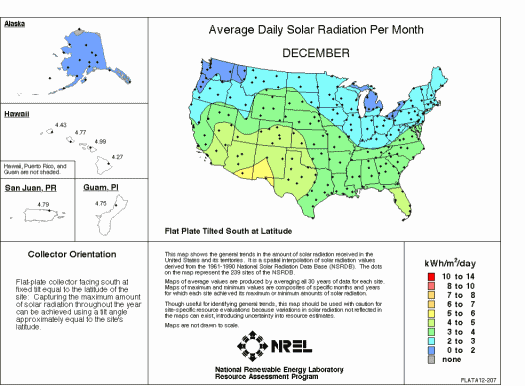


The sun  Always there; lots of energy.
Always there; lots of energy.
How many photons (energy) reach the surface of the Earth on average?
The energy balance in the atmosphere is qualitatively shown here:
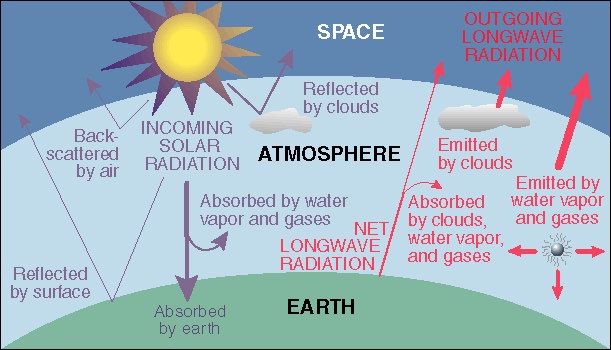
The main components in this diagram are the following:
What happens to the 69% of the incoming radiation that doesn't get reflected back:
 this has caused some to use stratospheric cooling as an argument against
the occurrence of global warming. The two are not connected
at all.
this has caused some to use stratospheric cooling as an argument against
the occurrence of global warming. The two are not connected
at all.
 Therefore it makes
absolutely no sense to put solar panels in orbit and then "beam"
the energy back to the surface.
Therefore it makes
absolutely no sense to put solar panels in orbit and then "beam"
the energy back to the surface.
Averaged over the entire, Earth =
164 watts per square meter over a 24 hour day  so the entire planet receives about 85,000 terrawatts of power
so the entire planet receives about 85,000 terrawatts of power  our current worldwide power use is about 14 TW (of which 3.5 are
required for the US alone). Since the Sun gives us 84,000, and we only need
15
our current worldwide power use is about 14 TW (of which 3.5 are
required for the US alone). Since the Sun gives us 84,000, and we only need
15  Is
this a solution?
Is
this a solution?
In principle, we would need 14 terrawatts (= 14 x 1012 watts) divided by 164 watts per square meter = 1011 square meters of collecting area.
But since our efficiency will only be about 10% (see more below), then we need 1012 square meters which is equal to one million square kilometers.
Now, suppose we could construct an equatorial grid with one station per
time zone. Then we would need 24 installations each of size 200 x 200
km.  This is formidable!
This is formidable!

and remember, little of the world current runs on
renewable energy sources:

There is a large amount of infrastructure (e.g. cost) required to convert from potential to deliverable energy.
 600 watts per sq. meter
600 watts per sq. meter
So over this 8 hour day one receives:
But to go from energy received to energy generated requires conversion of solar energy into other forms (heat, electricity) at some reduced level of efficiency.

We will talk more about PV cells in detail later. For now the only point to retain is that they are quite low in efficiency!
A typical household winter energy use, with electrical heating, is around 2000-3000 KWHs per month or roughly 70-100 KWH per day.
Assume our roof top area is 100 square meters (about 1100 square feet).
In the winter on a sunny day at this latitude (40o) the roof will receive about 6 hours of illumination.
So the incident solar energy over this 6 hour period is:
300 watts per square meter x 100 square meters x 6 hours
= 180 KWH (per day)  more than you need.
more than you need.
But remember the efficiency problem. We have to build a device to convert incident solar power into deliverable power. For now, again we don't care what the device is, only that is efficiency lies somewhere in the range below:
 9 KWH per day
9 KWH per day
 18 KWH per day
18 KWH per day
 36 KWH per day
36 KWH per day
At best, this represents 1/3 of the typical daily winter energy usage, and it assumes the sun shines on the rooftop for 6 hours that day.

With sensible energy conservation and insulation
and south facing windows, it's possible to lower your daily use
of energy by about a factor of 2. In this case, if solar shingles
become 20% efficient, then they can provide 50-75 % of your
energy needs.
These considerations suggest that solar PV rooftops (e.g., solar shingles) are likely a generically good idea.

What about the prospect of "solar farms" and remote power delivery?
The relative inefficiency can be compensated for with collecting area.
A site in Eastern Oregon receives 1200 watts per square meter of solar radiation in July. Assume that the solar panels are 10% efficient and that they are illuminated for 10 hours.
How many square meters would be required to power Eugene at 300 megawatts?
 Each square meter gives you 1200 x.1 = 120 watts
Each square meter gives you 1200 x.1 = 120 watts
 Remember, we want 300,000,000 Watts, not just 120.
Remember, we want 300,000,000 Watts, not just 120.
There are one million square meters in one square km.
So, each square km gives your 120 x 1,000,000 = 120 MW.
Therefore 2.5 square kilometers would be enough to power Eugene
Ten square kilometers would give you about 1200 MW  keep this as a figure of merit reference under optimum conditions.
keep this as a figure of merit reference under optimum conditions.
Now, of course, you wouldn't have continuous coverage. The individual collectors would have to be spaced out.

And so, in practice, the actual land use is about twice as great per MW generated. In addition, 1200 watts per square meter in July is about 2.5 times larger than the annual average. These considerations then produce the following rubric:
|
10,000 Megawatts of Average Annual Solar Power (when the sun is shining)
requires 500 square kilometers of collecting area (at 10% efficiency).
|
This is why any gain in efficiency is incredibly important.