For intelligent life to exist in the Universe, stable planets
must exist.
While there are strong theoretical reasons that to believe that solar system/planetary formation is a natural consequence of star formation, it wasn’t until 1995 that first bonafide extra solar planetary system was discovered.
Over the last 8 years, substantial progress has been made in
detecting the existence of other solar systems beyond our own.
As of September 17 2012, there are 834 known extrasolar
planets and more are being discovered each year at
the rate of over 100 per year.
Extrasolar Planetary
Status in near Real Time
first direct imaging of another planet
1st earth-size planet found from Kepler Spacecraft
Thousands of candidate planets
As mentioned before, planetary formation will occur after there
has been sufficient time for the Galaxy to be seeded with heavy
elements created through the process of stellar evolution and
supernova ejection.
Thus we expect to find planetary systems around stars that
have been created within the last few billion years because
in that case the protostellar cloud will have been enriched in
heavy elements. This expectation is clearly borne out by the
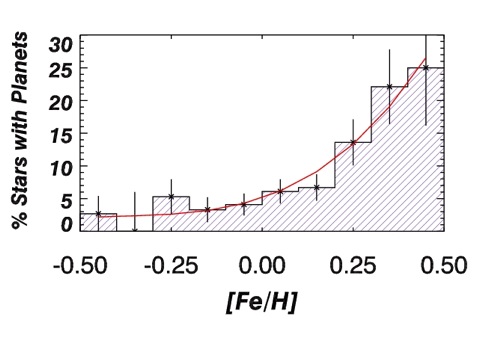
data (from Fischer and Valenti, Astrophysical Journal 2005, 622,1102 – in public domain):

In the above graph, the amount of stellar processing that has occurred is on the X-axis (encoded as [Fe/H] – the ratio of the abundance of Iron to the abundance of Hydrogen). Stars that have formed more recently out of the debris of supernova enriched material will have [Fe/H] values greater than zero. Those with [Fe/H] less than zero, represent stars that are less enriched than the Sun. The data above clearly show a strong increase the probability of a planetary system with increasing heavy element abundance. While this is not surprising, the above trend clearly shows that, as the heavy element abundance of the galaxy increases, it becomes more and more probable that newly formed stars will also have planets in orbit about them.
There are four principle techniques that astronomers have used to discover extra solar planetary systems:
2. Astrometric Technique - This works on the same principle as the Doppler Wobble except rather than measuring velocity variations in the star one tries to measure its change in position in the sky due to the star being gravitational perturbed by its planetary system. While more straightforward, in principle, than Doppler Wobble method, this technique requires exquisite precision in the ability to measure position and, as we have discussed in the parallax section, this is difficult to achieve due to the smearing effects of the Earth’s atmospheric motion.
3. Transit method – In some cases, our line of site to a nearby solar system is such that planets in that system periodically pass in front of the host star, thus causing a mini-eclipse. Recent improvement in precision measurements of the apparent brightness of stars now allows for these planetary caused mini-eclipses to be detected in some cases. Kepler spacecraft used this technique to find Earth-sized planets and has found this many planets.
4. Direct Optical Detection - Obviously this is the most straightforward way to detect planets but simply imaging nearby stars will not reveal planets because the light from the planet(s) is completely overwhelmed by the light from the star. Newly developed optical techniques, however, have discovered ways in which to mask that host star light to see if any residual light remains which would be due to the planets in orbit about it.
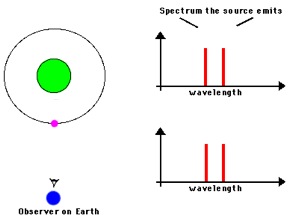
Consider the case of our Sun and Jupiter. Jupiter is sufficiently massive that it actually exerts a small but noticeable gravitational tug on the Sun. To an external observer, the Sun will be observed to have a small change in its radial velocity. Radial velocity refers to the motion that is either directly towards or away from an observer. Motion of an emitting source towards or away from an observer will change the wavelength/frequency of the radiation received by the observer relative to that emitted by the source. This is called the Doppler Effect or Doppler Shift. The Doppler shift can be easily explained via the diagrams below:

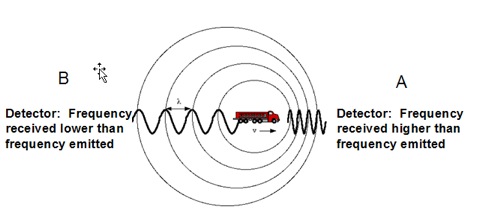
In the top diagram, the fire engine is not moving but nonetheless testing its siren for all to enjoy. In this case, the frequency that is detected (by your ear) is the same as the frequency that is emitted. But what happens when the fire engine is moving, from left to right, as illustrated in the bottom diagram. In this case, the sound waves are compressed in the direction of motion as the fire engine comes towards (blueshift) observer A – that observer hears a higher pitched siren. In the case of Observer B, the fire engine is moving away from them, along their direct line of site (e.g. radial motion) and the waves are elongated in this case (as the source is moving away from the observer - redshift) and the observer hears a lower frequency siren. The fire engine system is completely analogous to the case where a planet and its host star are in mutual orbit about their center of mass; As shown below, at certain times in the orbit the star will be moving directly towards the observer (blueshift) and at other times the star is moving directly away from the observer (redshift):
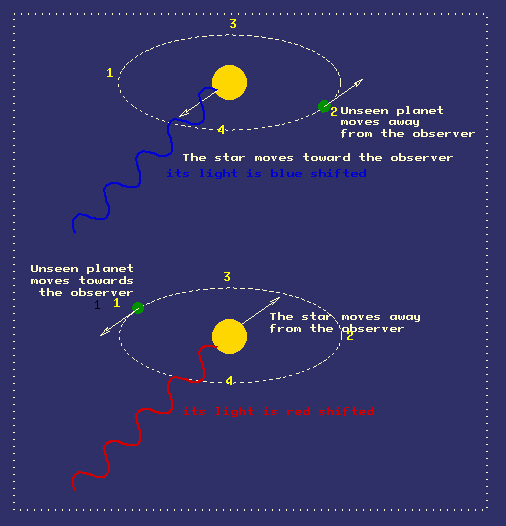
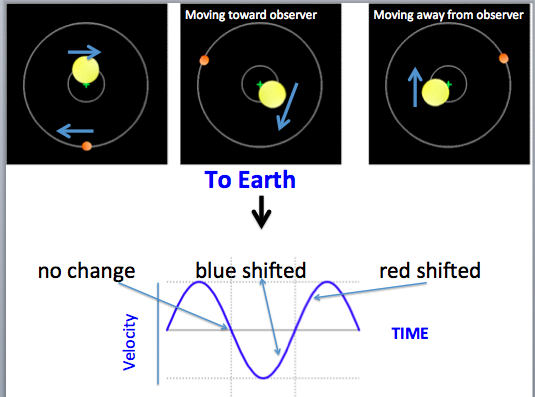
This change in the radial velocity of the star is called the “Doppler Wobble”. For the case of our solar system, Jupiter exerts a sufficient gravitational force on the Sun to produce a 12 meter/second perturbation in the Sun’s radial velocity. Thus, if an external observer were observing the sun and had an instrumental precision to detect a 12 meter/second variation, then that observer would deduce the presence of Jupiter. Key to this is having the instrumental precision needed to make this detection. For observers on the Earth, it wasn’t until circa 1995 that such instrumental precision became routine, and then the great planetary hunt was on. The period of variation in the Doppler Wobble will be directly determined by the orbital period of the planet. Thus a typical radial velocity curve (Doppler wobble curve) would look like the following:

The amplitude of this curve depends upon the mass and separation from the star of the perturbing planet. The period of the curve is the orbital period of the planet.
In this particular case, the orbital period is about 3 years and many orbital periods have been detected.
The amplitude (peak to trough variation) of this curve depends upon the mass and separation from the star of the perturbing planet. The period of the curve is the orbital period of the planet. In this particular case, the orbital period is about 3 years and many orbital periods have been detected, thus giving confidence of a real planetary detection. The vertical lines going through each data point represent the precision of that measurement – note that the post 1994 measurements all have much higher precision than the earlier ones.
Therefore, if we can monitor nearby stars for radial velocity variations at the level of a few meters per second, then we can potentially detect Jupiter mass planets in orbit about them. Until recently, however, this was easier said than done. To produce a detection, precision radial velocity observations are needed. Until about 1995, this was not possible with instrumentation. For instance, the typical precision was 25 meters per second, and such an instrument clearly would not detect a system like Jupiter and our Sun. In addition, one generally needs to observe a full orbital cycle to be sure that you have detected the presence of a perturbing planet. So, for instance, even an external observer was monitoring the radial velocity variation of our Sun over a 6 month period, they would not be able to detect the presence of Jupiter, since its orbital period is 5 years.
The race to find new planets officially began in October 1995 with the discovery of a Jupiter mass planet in the star 51 Pegasus. The details of that discovery, as well as an early example of using the Internet as a way to communicate scientific discoveries to the public, can be found at the original site: http://zebu.uoregon.edu/51peg.html (which has remained unchanged since its inception). Given the success achieved to date, it’s fairly clear that the excitement of the 51 Peg system has infused the astronomical community with a systematic and determined effort to find and characterize as many extra solar planetary systems as possible. As the precision of our measurements gets better, the ability to find lower mass planets increases and a recently completed survey down a the European Southern Observatory in Chile has successfully found 5 new planets around nearby, very low mass stars, of masses 4 to 25 times than of the Earth. These low mass planets were discovered primarily because they are at distances equivalent to the distance between Mercury (0.1 Earth masses) and the Sun, so the amplitude of their perturbation was fairly large.
For example, Jupiter has a mass of 316 Earth masses and is located at a distance of 5 AU from the sun (1 AU = 1 Astronomical Unit = distance from the Earth to the sun). The amplitude of the radial velocity variation on the host star essentially directly with the mass and also with the square root of the distance between the host star and the planet. For the case of the Jupiter-Sun system, the more exact perturbation in radial velocity of the sun is 12.7 meters/second.

Referring now to the above table:
• If your instrumental configuration were limited to 10 meters/second then only configurations 1 and 6 could be reliably detected.
• There is no chance of detecting configuration 4 with current instrumentation.
• 10-20 Earth Mass planets can only be detected if they are within 0.1-0.2 AU from the host star (the orbital distance of Mercury from the Sun is 0.1 AU)
• Configuration 2 is barely detectable with the best ground based instrumentation.
• Configurations 5 and 6 would require decades of observation before detected. Generally speaking you need to able to detect at least ¼ of an orbit with reliable velocity changes to have secure detection.
Therefore, the kinds of extra solar planetary systems that can be detected depend upon a) the instrumental precision and b) the time period of the observations relative to the intrinsic orbital period of the variation. As a result, certain kinds of configurations are more easily detected than other kinds of configurations. But this doesn’t mean that those other kinds of configurations don’t exist.