Diffraction of light occurs when a light wave passes by a corner or through an opening or slit that is physically the approximate size of, or even smaller than that light's wavelength. Diffraction means surface scattering that essentially creates new wave fronts that propogate in the same direction as the original incident light. This same principle applies to the light "rays" that are produced by some cloud formations.

Attenuation of Radiation by Direct Means in the Atmosphere:





For scattering, the details are all in the ratio of wavelength to scatter particle size. When the particles are very small relative to the wavelength then you have Rayleigh scattering which is strongly wavelength dependent (λ^-4). For instance, light (blue) at 4000 angstrom compared to light (red) at 7000 angstroms is satter (7/4)⁴ = 9 times more efficiently which of course is why the sky is blue:

Derivation of Rayleigh Scattering:

Start with a hydrogen atom (proton + electron) as the source of scattering of electromagnetic waves. Almost all of the scattering comes from the electron so we can just concentrate on the equation of motion for the electron when it encounters the incident wave.

We will assume a plane EM wave of some angular frequency ω which is polarized such that the associated electric field is parallel to the Z-axis. For this case we can write down:



We can then approximate the electron's equation of motion as

• The first term is just the force of electrostatic attraction between the electron and the proton.
  
  
• The second term is the perturbing force due to the incoming wave.
  
  

We are modeling this interaction now as a simple harmonic oscillator of frequency ω_o where ω_o can be thought of as a characterstic frequency that the atom emits after it is disturbed by the incoming plane wave. In the real world ω_o is a spectral line.

Solving for z:



In the dipole approximation, the power emitted per unit solid angle is given by



where a² representes the time-average square acceleration. We can use the equation of motion to compute a² for this case and that will clearly lead to a term of ω_o⁴.

Eventually if you did all the math and use the dipole moment for an electron and the derived classical electron radius to yield the thompsen cross section (σ_t) you would eventually get to this scaling expression for cases where ω_o >> ω



And this is what Rayleigh did 150 years ago.

In general scattering is characterized by a non-dimensional size parameter, x

• r = radius of the assumed spherical particle
• λ = wavelength of incoming light

This sets up these kinds of scattering reimes:



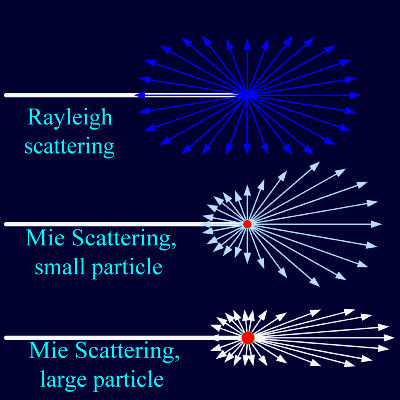
In addition the particle properties relative to the surrounding medium are important, particularly the complex refractive index.

Below is a convenient table of various kinds of atmospheric particles and their size and number concentration:



Refractive indices (measured at 589 nanometers, the peak wavelength of solar emission) for various different substances are given below and note that Carbon (usually in the form of soot) has a large value of the imaginary part of the refractive index so it is an effect absorber of a wave.



As we will see more below, Mie Scattering gets complicated and for large values of x there is very strong forward scattering (same direction as incoming light)

For cloud water droplets equal amounts of light as a function of wavelength are scattered in all directions so the cloud appears white. This is because the size distribution of the cloud droplets is quite uniform. At high altitudes this is done by small ice crystals - in fact this is why we know they are small, the clouds are white.

In general, scattering from particles is much stronger than that from molecules. Therefore particles (mostly from pollution and aerosols) become a very large component uncertainty in radiation models and climate change (discussed later). This is because the size distribution of particles is not well known and so the amount of forward scatter radiation is difficult to measure and has to be modelled.

The scattering efficiency of particles Q_s